Rates P6 2010 Kong Hwa CA1 Q9

A group of painters is painting the classrooms. If they paint 6 classrooms per day, they will be able to complete 1 day ahead of schedule. If they paint 4 classrooms per day, they will be 2 days behind schedule. How many classrooms are there?


                            6 x 1 (1 day earlier)
                              |   |
[   ][   ][   ][   ][   ][   ]
[   ][   ][   ][   ]       |
                       |       |Number of classrooms
                       4 x 2 (need 2 more days)

2 u  -->  4 x 2  +  6 x 1  =  14

1 u  -->  14 / 2 = 7 days

7  -  1  =  6

6  x  6  =  36 classrooms

[or 7  +  2  =  9
      9  x  4  =  36 classrooms]

There were 36 classrooms.

Decimal P6 2010 Pei Hwa CA1 Q18

7 apples cost $4.00.

9 oranges cost $11.00.
James bought a total of 1000 apples and oranges for $999.00.
How many apples and how many oranges did he buy?


7 A  +  9 O  --> 1000  Use 7 A and 9 O for the number of apples and oranges respectively to avoid handling fractions or decimals

4 A  +  11 O  -->  999

28 A  +  36 O  -->  4 x 1000 = 4000

28 A  +  77 O  -->  7 x 999 = 6993

41 O  -->  6993  -  4000  =  2993

9 O  -->  9/41  x  2993  =  657 oranges

7 A  -->  1000  -  657  =  343 apples


He bought 343 apples and 657 oranges.

Percentage P6

In a primary school, 50 pupils were surveyed on the pets they liked. 80% of the pupils liked dogs, 50% of them liked birds and 34% of them liked rabbits. The survey results were that 1 out of every 5 pupils did not like any of the animals. What is the least number of pupils who liked all 3 types of animals. [Ans : 2]


1/5 x 50 =10 -- did not like pets


100% --> 50

80% --> 80/100 x 50 = 40 -- like dogs

50% --> 50/100 x 50 = 25 -- like birds

34% --> 34/100 x 50 = 17 -- like rabbits

25 + 17 - 40 = 2 pupils   Least overlap between those who like birds and rabbits

The least number of pupils who liked all 3 types of animals is 2.

Whole Number P6

Wilson purchased a certain number of wallets at $6 each. If he had purchased those at $8 each, he would have purchased 20 fewer wallets. How much money did he have? [Ans : $480]


6 : 8 = 3 : 4         Ratio of the number is inversely proportional to the price

1 u --> 20

4 u --> 4 x 20 = 80       Note: 4 u at $6 (3 u at $8) [inverse proportion]

6 x 80 = $480

He had $480. 

Percentage P6

Benny spent 15% of his salary on food and $440 on camera. Then he spent 30% of the remainder on clothes and saved the rest of his salary. He found that the amount he spent on clothes was $120 more than the amount he had spent on food.
(a) How much did he spend on food? Ans: $360
(b) How much did he save? Ans: $1120


30% r --> 15% + $120

70/30 x 15% = 35%

70/30 x 120 = $280

70% r --> 35% + $280

100% - 15% - 15% - 35% = 35%

35% --> 440 + 120 + 280 = $840

15% --> 15/35 x 840 = $360

a) He spent $360 on food.


840 + 280 = $1120

b) He saved $1120.

Percentage P6

If Larry sells his toy at a discount of 20%, he can still make a gain of $150. If he sells it at a 45% discount, he will lose $325. How much did Larry pay for his toy?

80%  -->  1 u  +  $150

55%  -->  1 u  -  $325

80%  -  55%  -->  150  -  (- 325)

25%  -->  150  +  325  =  $475

80%  -->  80/25  x  475  =   $1520

1520 -  150  =  $1370

It was $1370.

Ratio P6

Larry, May and Nora shared the cost of a meal. The ratio of the amount paid by Larry to the total amount paid by May and Nora is 2 : 3 while May’s share was 20% of the total amount paid by Larry and Nora. Given that Nora paid $84 more than May, how much did the meal cost?


L : M + N  =  2  :  3
                  =  12  :  18  [x 6 to get total of same total of 30]

M : L + N  =  20  :  100  
                  =  1  :  5
                  =  5  :  25   [x 5 to get same total of 30]

30 - 12 - 5 = 13

L : M : N = 12  :  5  :  13

13 - 5 = 8

8 u  -->  $84

30 u  -->  30/8  x  84  =  $315

It was $315.

Ratio P6

Ali, Bill and Cindy had a total of 450 apples. The ratio of the number of apples Bill had to the number of apples that Cindy had was 2 : 5. After Ali and Bill had given away ½ of their apples, the children had 300 apples left. How many apples did Bill have at first?

A [  ][  ]                  ]

B [][][][]                 ]  450

C [][][][][][][][][][]  ]

A [  ]                      ]

B [][]                      ]  300

C [][][][][][][][][][]  ]

300 x 2 = 600

10 u --> 600 - 450 = 150

4 u  -->  4/10  x 150  =  60 apples

Bill had  60 apples  at first.

Circle P6 2010 SA2 ACS P2 Q14

pi  x  30 = 94.248



180/360  =  1/2

3  -  1/2  =  2 1/2

2 1/2  x  94.248  =  235.62 cm2 (2 dp)

The shaded area is 235.62 cm2.

Percentage P6 2010 SCGS P2 Q13

Terry bought a vase which he decides to sell. If he sells the vase at a discount of 20%, he makes a gain of $150. If he sells it at a 45% discount, he loses $325. How much did Terry pay for his vase? [4 marks][Answer given: $1370]



100%  -  20%  =  80%

80%  -->  Cost  +  $150


100%  -  45%  =  55%

55%  -->  Cost  -  $325


80%  -  55%  -->  150  +  325

25%  -->  $475

80%  -->  80/25  x  475  =  $1520

Cost --> 1520  -  150 =   $1370


The cost of the vase is  $1370.

Circle P6 2010 SA2 SCGS P2 Q18

[2 marks for (a) and 3 marks for (b)]{Answers given: 116 cm and 364.42 cm (correct to 2 decimal places)]


43  x  2 =  86  cm

86  x  2  =  172  cm

28  x  2  =  56  cm

172  -  56  =  116  cm

a) The length of SR is  116  cm.


86  -  56  =  30  cm

pi  x  30  =   94.247 cm 

2  x  pi  x  43  =  20.177  cm 

94.247  +  20.177  =  364.42 cm (2 dp)


The perimeter is 364.42 cm.

MGS 2010 P6 Prelim P2 Q11:

Ellen and Lenny have some sweets. If Ellen gives away 12 sweets, the number of sweets Ellen has is 13/24 of the total number of sweets that both of them have. If Lenny gives away 12 sweets, the number of sweets Lenny has is 3/8 of the total number of sweets that both of them have. How many sweets do they have altogether? [4 marks][Answer given: 156 sweets]


24  -  13  =  11

E  --  13 u  +  12

L  --  11 u


8  -  3  =  5

L  --  3 p  +  12

E  --  5 p


11 u  -->  3 p  +  12

13  u  +  12  -->  5 p


15 p  -->  55 u  -  12 x 5  =  55 u  -  60

15 p  -->  39 u  +  12 x 3 =  39 u  +  36


55 u - 60  -->  39 u  +  36


16 u  -->  36  +  60  =  96

1 u  -->  96 / 16 = 6

24 u  -->  24  x  6  =  144

144  + 12  =  156  sweets

They have  156  sweets altogether.

MGS 2010 P6 Prelim P2 Q17:

A tank contains some water up to a height of 10 cm. When 4 identical marbles are put into the tank, the water level rises by 8 cm. One marble is then removed from the tank and a metal block is put into the tank. The water level increases to 22 cm.
(a) Find the ratio of the volume of 1 marble to the metal block. [2 marks]
(b) If the base area of the tank is 450 cm², how much more is the volume of the metal block than that of two marbles? [3 marks]
[Answer given: (a) 1 : 3   (b) 900 cm³]



4 marbles  -->  8 cm

1 marble  -->  1/4  x  8  =  2 cm

8  -  2  =  6  cm

Metal block  -->  22  -  10  -  6 =  6 cm

2 : 6 = 1 : 3      Since base area of tank is the same

a) The ratio of the volume of 1 marble to the metal block is 1 : 3.


6  -  2 x 2  =  2 cm

450  x  2  = 900 cm3

b) The volume of the metal block is  900 cm3  more than 2 marbles.

An apple a day will keep the doctor away

In the morning, James ate an apple which his mother had just bought from the market. He then complained that the apple was neither sweet nor juicy. So his mother decided to put the remaining apples into the refrigerator.

In the afternoon, when James ate another apple which his mother had bought that morning, do you think he would find the apple sweet or juicy? Explain your answers.

Time P5 2009 SA2 RGS P1 Q26

Ali was given 0.18 litres of cough mixture by the doctor. He had to take 10 ml of it every 4 hours. How many days would he take to finish the cough mixture?


180 / 10 = 18 times

18 - 1 = 17      Exclude the 1st time he took the cough mixture as time is 0 h

17 x 4 = 68 hours

68/24 = 2 5/6 days

MATHS PSLE P1 Q1 - 15

1) 3
2) 1
3) 1
4) 3
5) 3

6) 4
7) 3
8) 1
9) 2
10) 2

11) 4
12) 4
13) 2
14) 1
15) 4

Whole Number PSLE 2010 P1 Q16

16. 9cm

Angle PSLE 2010 P1 Q17

17. 113°

Fraction PSLE 2010 P1 Q18

18.  Express 3.44 as a mixed number in the simplest form.



3.44 = 3 44/100
        = 3 22/50
        = 3 11/25

Fraction PSLE 2010 P1 Q19

19. Find the value of 2/3 + 4/7. Give your answer as a mixed number in the simplest form.


2/3 x 7/7  +  4/7 x 3/3  =  14/21  +  12/21
                                    =  26/21
                                    =  1 5/21

Decimal PSLE 2010 P1 Q20

20. Find the value of 5 ÷ 8. Express your answer as a decimal.


5/8 x 125/125 = 625/1000
                       = 0.625


    0.625
   ______
8 | 5.000
  - 4 8
 --------
       30
     - 24
    ------
         60
      - 56
       ------
           40
         - 40
          ----
             0
          ----

Decimal PSLE 2010 P1 Q21

21. Find the value of 0.16 x 40.  Answer:　6.4
 
 
0.16  x  40  =  1.6  x  4
                   =   6.4

Volume PSLE 2010 P1 Q22

22. What is 1.55 litres in millilitres?




1.55 x 1000  =  1550 ml

Decimal PSLE 2010 P1 Q23

23. John and Ken went to a funfair. John spent $25 and Ken spent 80¢ less than John. How much money did they spend at the funfair altogether?


25  -  0.80  =  24.20

24.20  +  25  =  $49.20

Fraction PSLE 2010 P1 Q24

Mr Tan took his family to the zoo. They were in the zoo from 9.10 a.m. to 3.10 p.m. What fraction of the 24-hour day did Mr Tan and his family spend in the zoo? Give your answer in the simplest form.


              3 h             3 h
    |---------------|--------------|
9.10 am      12.10 pm      3.10 pm




It was 1/4 of the 24-hour day.6/24 = 1/4

Percentage PSLE 2010 P1 Q26

26.  A school conducted checks on its Primary 1 pupils' eyesight from Monday to Thursday. Each of them had their eyes checked on one of the four days. The bar graph below shows the number of pupils that were checked on each day.


Bar graph:

Y-axis: Number of pupils (intervals of 20 and scale from 0 to 200)

X-axis: Monday, Tuesday, Wednesday and Thursday

Monday: 180

Tuesday: 120

Wednesday: 200

Thursday: 100

All columns in the graph are shaded.

What percentage of the Primary 1 pupils had their eyes checked on Monday?



180  +  120  +  200  +  100  =  600


180/600  x  100%  =  30%

Whole Number PSLE 2010 P1 Q29

Ans: 60

Fraction PSLE 2010 P1 Q30

30.  In the diagram below, ACEG and BDFH are squares. AB, CD, EF and GH are of the same length. The ratio of AB: BC is 2:1.

What fraction of the square ACEG is shaded?


3  x  3  =  9

4  x  1/2  x  1  x  2  =  4

9  -  4  =  5

5/9

Whole Number PSLE 2010 P2 Q1

1. Write down all the common factors of 12 and 18.


1 x 12    1 x 18


2 x 6       2 x 9


3 x 4      3 x 6


The common factors are 1, 2, 3, 6.

Compass PSLE 2010 P2 Q2

The square grid below shows the plan of a playground. The bench is north of the toy car.

(a) In what direction is the swing from the see-saw?

(b) The town council wants to plant a tree in the playground.

The location of the tree is to be north of the swing and south-west of the slide. Put a tick (√) in the square where the tree will be planted.


a) West

b) As shown in the diagram √

Decimal PSLE 2010 P2 Q3

Meijun had some money. She used 1/4 of it on a watch and 1/6 of it on a gift. The watch and the gift cost $133.50 altogether.How much money had she left?


1/4 x 3/3 = 3/12

1/6 x 2/2 = 2/12

3 + 2 = 5

12 - 5 = 7

5 u  -->  133.50

7 u  -->  7/5 x 133.50 =  $186.90


She had $186.90 left.

Area & Perimeter PSLE 2010 P2 Q4

In the figure below, AB=7 cm, BC=9 cm, CD=3 cm and DA=11 cm. Angle ABC and Angle CDA are right angles.

Find the area of the figure ABCD.


1/2 x  9 x 7  =  31.5


1/2 x 11 x 3  =  16.5


31.5  +  16.5  =  48  cm2

It is  48  cm2.

Speed PSLE 2010 P2 Q5

Faizal and Gary ran in a race. When Gary had completed the race, Faizal had only run 5/8 of the distance. Gary's speed was 75 m/min faster than Faizal's speed. Both of them did not change their speeds throughout the race. What was Faizal's speed in m/min?


3 u  -->  75 m/min

5 u  -->  5/3  x  75  =  125  m/min

It was  125  m/min.

Algebra PSLE 2010 P2 Q6

6.  Alan had 1.2 m of wire. He used some of it to make the figure shown below.


A pentagon is given, labelled from left to right and base: k cm, k cm, k cm, (k + 8)cm and (2 k - 5) cm.

(a) How much of the wire did Alan use to make the figure?
      Leave your answer in the simplest form in terms of k.

(b) If k=15, how much of the wire was not used to make the figure?


k  +  k  +  k  +  (k + 8)  +  (2 k - 5)  = (6 k  +  3) cm

a) Alan used (6 k + 3) cm.



6 x 15  +  3  =  93


120  -  93  =  27  cm

It was  27  cm.

Average PSLE 2010 P2 Q7

The table below shows the number of books read by each pupil in a class of 30 pupils. One of the numbers in the table is covered by an ink blot.


---------------------------------------------------------------
| Number of books read by each pupil   |   0   |   8   |  ??  |
---------------------------------------------------------------
| Number of pupils                                 |  10  |  15  |   5   |
---------------------------------------------------------------

The average number of books read by the pupils in the class is 6.


What is the number covered by the ink blot?


30  x  6  =  180


15  x  8  =  120


180  -  120  =  60


60  ÷  5  =  12

It is  12.

Decimal PSLE 2010 P2 Q8

8. A jar filled with 40 identical screws weighs 1.4 kg. The same jar when filled with 20 identical nails weighs 500 g. The mass of each screw is twice the mass of each nail. What is the mass of the empty jar?


J + 80 u -- > 1.4


J + 20 u -- > 0.5



60 u --> 1.4 - 0.5 = 0.9


20 u --> 20/60 x 0.9 = 0.3



J  -->  0.5 - 0.3 = 0.2 kg


The mass of the empty jar is 0.2 kg.

Angle PSLE 2010 P2 Q9

9.  In the figure below, ABCD is a trapezium. E is a point on AD such that AB=BE. Angle BCD=62⁰ and angle CDE=110⁰

Find angle EBC.

180  -  110  =  70

180  -  70  -  70  =  40

180  -  62  =  118

118  -  40  =  78°

It is 78°.

Area & Perimeter PSLE 2010 P2 Q10

10. ADEF is a rectangular cardboard with AF = 7 cm. Two quarter circles have been cut from it as shown below. The remaining cardboard, which is the shaded part, has an area of 56 cm².
Using π=22/7, find the length of BC.

1/2  x  22/7  x  7  x  7  =  77

77  +  56  =  133

133/7  =  19

19  -  7  -  7 =  5 cm

It is 5 cm.

Whole Number PSLE 2010 P2 Q11

Sam packed 320 marbles into four boxes, labelled A, B, C and D. Box A had the most number of marbles and box D had the least. The difference in the number of marbles between Box A and the number of marbles in the other three boxes were 8, 13 and 19.
How many marbles were there in Box D?

D []<----- 19 ----->    ]
C [][ 6 ]<-- 13 --->    ]
B [][ 11  ]<-- 8 -->    ] 320
A [][        19        ]   ]

4 u  -->  320  - 6 - 11 - 19 = 284    Small unit -- D

1 u --> 284 / 4 = 71

It is 71 marbles.


Alternative method (model drawing is actually not required)
------------------------
A [                             ]      ]
B [                 ]<-- 8-->      ]
C [             ]<-- 13 --->     ]  320
D [     ]<---- 19 -------->     ]

4 u  -->  320  +  8  +  13  +  19  =  360     Big units -- A 

1 u -->  360 / 4  =  90

90  -  19  =  71

It is  71  marbles.

Whole Number PSLE 2010 P2 Q12

A group of girls shared some sweets among themselves. They tried taking 11 sweets each, but found that the last girl had only 6 sweets. When each girl took 8 sweets, there were 25 sweets left over. How many sweets were there altogether?



                                                            [     ]   -- 1 u
[    ][    ][    ][    ][    ][    ][    ][    ][    ][    ][6][5]
[    ][    ][    ][    ][    ][    ][    ][    ][       25   ]

3 u  -->  25  +  5  =  30

1 u  -->  30/3 = 10

11 u  -->  11 x 10  =  110

110  - 5  = 105  sweets

There are 105  sweets.


Alternative method
-------------------------
11 x (n - 1)  +  6  -->  8  x  n  +  25

3 n  -->  25  -  6  +  11  =  30

n  -->  30/3  =  10

8  x  10  +  25  =  105 sweets

There are  105  sweets.

Angle PSLE 2010 P2 Q13

In the figure below, ABC is a triangle. X, Y and Z are points on the triangle such that AX=AY and YB=ZB.

If angle AYZ=104⁰ and angle XYB=107⁰, find angle ZCX.

180  -  104  =  76

180  -  76  -  76  =  38

180  -  107  =  73

180  -  73  -  73  =  34

180  -  28  -  34  =  118⁰

It is  118°.

Alternative solution provided by P6 pupil

107° + 104°  =  211°

211°  -  180°  =  31°

360°  -  31°  -  107°  -  104°  =  118°

Percentage PSLE 2010 P2 Q14

14.  Mrs Cheng bought a bag and a pair of shoes at a discount. She spent a total of $57.60 on these two items. She spent $9.60 more on the shoes than on the bag.

(a) How much did she spend on the shoes?

(b) The total discount given for the two items was $22.40. She was given a 25% discount for the bag. What was the percentage discount given for the shoes?


S  +  B   --> $57.60

S  -  B  -->  $9.60

2 S  -->  57.60  +  9.60  =  $67.20

S  -->  67.20 / 2  =  $33.60

a) She spent $33.60 on the shoes.


B  -->  57.60  -  33.60  =  $24

75%  -->  $24

25%  -->  25 / 75 x  24  =  $8

22.40  -  8  =  $14.40

33.60  +  14.40  =  $48

14.40 / 48  x  100%  = 30%

b) The percentage discount given for the shoes was 30%.

Whole Number PSLE 2010 P2 Q16

16.  Nurul and Peili went shopping together with a total sum of $60. Nurul spent twice as much as Peili. The amount Peili had left was $7 more than what she had spent. She had twice as much money left as Nurul.
(a) How much money did Peili spend?
(b) How much money did Nurul have at first?


N [][][][]  [][3.50]                 }
P [][]        [][][      7       ]       } $60

9 u  -->  60  -  7  -  3.50  =  49.50

1 u  -->  49.50 / 9  =  5.50

2 u  -->  2  x  5.50  =  $11

a) Peili spent  $11.


5 u  -->  5  x  5.5  =  27.50

27.50 +  3.50  =  $31

b) Nurul had  $31 at first.

Challenging PSLE 2010 P2 Q17

Structure Number  Number of Rods  Height (cm)
--------------------------------------------------------
            1                                 12   15      3                              
            2                   12 + 8 = 20             3
            3                   20 + 8 = 28             6   Pattern starts
            4                   28 + 5 = 33             6   from Structure 3
            5                   33 + 8 = 41             9    onwards
            6                   41 + 5 = 46             9
----------------------------------------------------------

H (119)  -->  3  x  (119 + 1) / 2 = 180 cm


b) It is  180  cm.


R (119)  -->  20  +  8  x  (119 - 1)/2 + 5 x (118 - 2)/2 = 782 rods


c) There are  782  rods.


Alternative solution for (c)
-------------------------------
Adjusted Structure 1  -->  20  -  5  =  15

15 , 20 , 28 , 33 , 41   (+ 5 , + 8, + 5, + 8)

R (119)  -->  15  +  (119 - 1)/2  x (8 + 5)  =  782 rods

c) There are 782 rods.

Average P5 2009 SA2 Nanyang P2 Q14

Joanne bought 7 balloons at the carnival. After that she bought another 4 balloons at $9.80 each the average cost of the balloons increased by $0.60. What was the total cost of the balloons? [4 marks]


9.80 x 4 = 39.20

0.60 x 7 = 4.   Total increase of 7 balloons

39.20 - 4.20 = 35.00    Total of 4 balloons after decrease (inrease in 7 balloons)

35.00 / 4 = 8.75   Average

8.75 x 11 = $96.25

It was $96.25.

Australian Mathematics Competition Junior Q26

An ascending number is one in which each successive digit is greater than the one before. Find the 3-digit descending number which is the square of an ascending number?


29  x  29  =  841 descending

Volume P6 2010 SA2 Pei Chun P2 Q15

36 x 36 x 36 = 46656 cm3

46656/(72 x 24) = 27 cm

7 u  -->  27 + 15 = 42

8 u  -->  8/7  x 42 =  48 cm

72 x 24 x 48 = 82944 cm3

                    = 82.944 litres

It was 82.944 litres.

Percentage P6 2010 SA2 NY P2 Q1

Challenging P6 2010 SA2 NY P2 Q2

Fraction P6 2010 SA2 NY P2 Q3

Volume P6 2010 SA2 NY P2 Q4

Area & Perimeter P6 2010 SA2 NY P2 Q5

Whole Number P6 2010 SA2 NY P2 Q6

Decimal P6 2010 SA2 NY P2 Q7

Angle P6 2010 SA2 NY P2 Q8

Percentage P6 2010 SA2 P2 NY P2 Q9

Speed P6 2010 SA2 Nanyang P2 Q10

Kingsley planned to cycle from Town P to Town Q. If he were to cycle at 10 km/h, he would reach Town Q at 7.45 p.m. If he were to cycle at 12 km/h, he would reach Town Q at 7.15 p.m. What was the distance between Town P and Town Q? [3 marks][Answer given: 30 km]


                                                          1/2 h
 ---> 10 km/h                         7.15 pm            7.45 pm
 |-------------------------------|-----------------|
P                                                                       Q
 -----> 12 km/h                                             7.15 pm

10 x 1/2 = 5 km     Difference in distance

12 - 10 = 2 km/h     Difference in speed

2 km --> 1 h

5 km --> 5/2 x 1 = 2 1/2 h       Total time to cause the difference in distance

12 x 5/2 = 30 km    Note: use 12 km/h (For 10 km/h, the journey is not completed yet

It was 30 km.

Volume P6 2010 SA2 Nanyang P2 Q 11

40 x 20 x 10 = 8000   Amount of water upto bottom of cube

12000 - 8000 = 4000 cm3   Amount of water from above bottom of cube

40 x 20 = 800

10 x 10 = 100

800 - 100 = 700 cm2   Area of water just above bottom of cube

4000 / 700 = 5.71  Height of water above bottom of cube

5.71 + 10 = 15.71 cm    Height of water

It was 15.71 cm.

Angle P6 2010 SA2 NY P2 Q12

Decimal P6 2010 SA2 Nanyang P2 Q13

Quiny and Mandy saved $5748.40 altogether. 0.4 of Quiny’s savings was $431.50 more than 0.2 of Mandy’s savings. How much more money than Quiny did Mandy save? [4 marks][Answer given: $477.80]


Quiny's money --> 10 Q
Mandy's money --> 10M
4 Q --> 2 M + $431.50

2 M --> 4 Q - $431.50

10 M --> 20 Q - 5 x 431.50 = 20 Q - $2157.50


10 Q + 10 M --> $5748.40

30 Q --> 5748.40 + 2157.50 = 7509.90

10 Q --> 7509.90/3 = $2635.30

10 M --> 2 x 2635.30 - 2157.50 = $3113.10

10 M - 10 Q --> 3113.10 - 2635.30 = $477.80

It was $477.80.


Model Drawing
------------------

431.50 / 2 = $215..75   0.4 = 4/10 = 2/5

Compare 2 units of Q (total 5 units) with 2 units of M (total 10 units)
Q  [][215.75][][          ][][           ][][            ][][             ]   ] $5748.40
M [][][][][][][][][][]                                                            ]

215.75 x 5 = 1078.75

15 u --> 5748.40 - 431.50 = 4669.65

5 u --> 5/15 x 4669.75 = 1556.55

1078.75 - 1556.55 = $477.80

It was $477.80.

Average P6 2010 SA2 NY P2 Q14

Challenging P6 2010 SA2 Nanyang P2 Q15

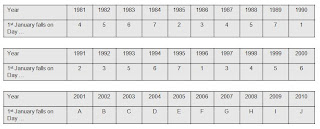
The table below shows the day of the week that 1st January falls on from the year 1981 to 2000. Note that Day 1 of a week is a Monday and Day 7 is Sunday. There are 31 days in the month of January.

a) What number does the letter C represent ? (Ans : 3)

b) What number does the letter F represent ? (Ans : 7)

c) Which day of the week will 1st February 2012 fall on ? (Ans : Wednesday)

2003 - 1981 = 22 years

22/4 = 5 leap years (max)   Leap year --> 366 days

22 x 365 + 5 =8035 days    Account for leap years

8035 + 4 = 8039     Since 1 Jan 1981 is 4 (Thursday).

8039/7 = 1148 r 3

a) It was 3.

2006 - 1981 = 25 years

25/4 = 6 leap years (max)

25 x 365 + 6 + 4 = 9135

9135/7 = 1305 or 1304 r 7   Need to express with a remainder

b) It was 7.

2012 - 1981 = 31 years

31/4 = 7 leap years (max)

31 x 365 + 31 + 7 + 4 = 11357 days

11357/7 = 1622 r 3

3 --> Wednesday

c) It is Wednesday.