A seamstress had some white and some black buttons.60% of the buttons were white.He bought another 120 buttons.Now the percentage of white buttons became only 40% of the total.How many white buttons did he have at first?
W B
---------
3 : 2 ---- 5 units
+ 120
---------
2 : 3 ----- 5 parts
---------
5 p --> 5 u + 120
1 p --> 1 u + 120/5 = 1 u + 24
2 p --> 2 u + 2 x 24 = 2 u + 48
3p --> 3u + 3 x 24 = 3 u + 72
3 u + ? --> 2 u + 48
2 u + (120 - ?) --> 3 u + 72
Both equations will end up with 1 u --> 48 -
Solution 1
-----------
W [][][][][][]
B [][][][][][][][][]
5 u --> 120
1u --> 120 /5 = 24
W: 6 u --> 6 x 24 = 144 white buttons.
Solution 2
------------
W [][][][][][][][]
B [][][][][][][][][][][][]
10 u --> 120
1u --> 120/10 = 12
W: 6 u --> 12 x 6 = 72 white buttons.
Solution 3
-----------
W [][][][][][][][][][][][][][]
B [][][][][][][][][][][][][][][][][][][][][]
15 u --> 120
1 u --> 120/15 = 8
W: 12 u --> 12 x 8 = 96 white buttons.
etc.......
Monday, August 30, 2010
Sunday, August 29, 2010
Friday, August 27, 2010
Speed P6
Jack cycles to his friend's house and then returns home by the same route. He always cycles at 4 km/h when going uphill, 12 km/h when going downhill and 6 km/h when on level ground. If his total cycling time is 2h 20mins, what is the distance he cycles in km?
D1 - uphill, D2 - downhill, D3 - level ground
Time from Home to Friend's house --> D1/4 + D2/12 + D3/6
D3 - level ground, D2 - uphill, D1 - downhill
Time from Friend's house to Home --> D3/6 + D2/4 + D1/12
Total time --> D1 (1/4 + 1/12) + D2 (1/12 + 1/4) + D3 (1/6 + 1/6)
= 1/3 D1 + 1/3 D2 + 1/3 D3
= 1/3 D
1/3 D --> 2 20/60 = 7/3
D --> 3 x 7/3 = 7 km
7 x 2 = 14 km
The distance he cycles is 14 km.
D1 - uphill, D2 - downhill, D3 - level ground
Time from Home to Friend's house --> D1/4 + D2/12 + D3/6
D3 - level ground, D2 - uphill, D1 - downhill
Time from Friend's house to Home --> D3/6 + D2/4 + D1/12
Total time --> D1 (1/4 + 1/12) + D2 (1/12 + 1/4) + D3 (1/6 + 1/6)
= 1/3 D1 + 1/3 D2 + 1/3 D3
= 1/3 D
1/3 D --> 2 20/60 = 7/3
D --> 3 x 7/3 = 7 km
7 x 2 = 14 km
The distance he cycles is 14 km.
Challenging P6
A pair of hamsters can give birth to a pair of baby hamsters every month. Each pair of baby hamsters needs 1 month to mature and then this pair of hamsters will in turn give birth to a pair of baby hamsters every month. How many pairs of hamsters are there after 6 months? (4 marks)
The last digit represents the number of pairs of hamsters born. The front digits/alphabet represent the parents. Remember to add 1 (S).
The last digit represents the number of pairs of hamsters born. The front digits/alphabet represent the parents. Remember to add 1 (S).
Wednesday, August 25, 2010
Challenging P5 Chong Fu
Nicholas has two 6-sided dice. One dice has numbers 1 ,3, 5, 7, 11 and 13 while the other dice has numbers 4, 8, 18, 24, 30 and 36. The dice are tossed together and their values added.
a)What fraction of the outcomes adds up to a sum divisible by 7?
b)What fraction of the outcomes adds up to a sum divisible by 3?
There are 36 outcomes (6 x 6 = 36)
Multiples of 7 --> 6 outcomes
(3 + 4, 3 + 18, 5 + 30, 11 + 24, 13 + 8, 13 + 36)
6 ÷ 36 = 1/6
a) Fraction of outcomes of sum divisble by 7 is 1/6.
Multiples of 3 --> 9 outcomes
(1 + 8, 3+ 18, 3 + 24, 3 + 30, 3 + 36, 5 + 4, 7 + 8, 11 + 4, 13 + 8)
9 ÷ 36 = 1/4
b)Fraction of outcomes of sum divisible by 3 is 1/4.
a)What fraction of the outcomes adds up to a sum divisible by 7?
b)What fraction of the outcomes adds up to a sum divisible by 3?
There are 36 outcomes (6 x 6 = 36)
Multiples of 7 --> 6 outcomes
(3 + 4, 3 + 18, 5 + 30, 11 + 24, 13 + 8, 13 + 36)
6 ÷ 36 = 1/6
a) Fraction of outcomes of sum divisble by 7 is 1/6.
Multiples of 3 --> 9 outcomes
(1 + 8, 3+ 18, 3 + 24, 3 + 30, 3 + 36, 5 + 4, 7 + 8, 11 + 4, 13 + 8)
9 ÷ 36 = 1/4
b)Fraction of outcomes of sum divisible by 3 is 1/4.
Labels:
M Challenging P5,
M Challenging P5 CF,
M P5 CF
Sunday, August 1, 2010
NMOS 2010 Q2
Q2. Adrian, Bryan, Charles, David, Elvin and Fedric played against one another in a singles tournament such that each one of them played every other player only once, and none of the games ended with a draw. If Adrian won 4 games, Bryan won 3 games, Charles won 2 games, David won 2 games and Elvin won 2 games, how many games did Fedric win?
6 x 5 / 2 = 15
15 - 4 - 3 - 2 - 2 - 2 = 2 games – won by F
6 x 5 / 2 = 15
15 - 4 - 3 - 2 - 2 - 2 = 2 games – won by F
NMOS 2010 Q3
Q3. At 6 a.m. in the morning, Andrew (at Town A) and Benjamin (at Town B) were travelling in opposite directions towards each other. They met each other along the way at 12 noon. Andrew reached Town B at 4 p.m. If they travelled with different constant speeds, how long (in hours) did Benjamin take to travel from Town B to Town A?
6 h : 4 h
A -- A (6 a.m.) --------------- M (12 noon) -------------- B (4 p.m.)
B – A (?) ---------------------- M (12 noon) -------------- B (6 a.m.)
6/4 x 6 : 6 h
= 9 h
9 + 6 = 15 h
6 h : 4 h
A -- A (6 a.m.) --------------- M (12 noon) -------------- B (4 p.m.)
B – A (?) ---------------------- M (12 noon) -------------- B (6 a.m.)
6/4 x 6 : 6 h
= 9 h
9 + 6 = 15 h
NMOS 2010 Q4
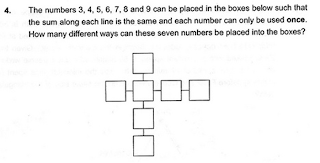
[ ]
[ ][ ][ ][ ]
[ ]
[ ]
3 + 4 + 5 + 6 + 7 + 8 + 9 = 42 -- even number
So thee number in the box at the intersection will have to be an even number -- 4, 6 or 8.
42 + 4 = 46
46 / 2 = 23
4 + 6 + 5 + 8 = 23 4 + 3 + 7 + 9 = 23
Digit 4 is fixed, so only 1 way.
5, 6 and 8 --> 3 x 2 x 1 = 6 ways
3, 7 and 9 --> 3 x 2 x 1 = 6 ways
5, 6, 8 and 3, 7, 9 can be switched, i.e. vertical becomes horizontal and horizontal becomes vertical
So total number of ways --> 1 x 6 x 6 x 2 = 72
42 + 6 = 48
48/2 = 24
6 + 7 + 3 + 8 = 24, 6 + 4 + 5 + 9 = 24
Number of ways --> 72 ways
42 + 8 = 50
50 / 2 = 25
8 + 7 + 4 + 6 = 25, 8 + 3 + 5 + 9 = 25
Number of ways --> 72 ways
Total number of ways --> 72 x 3 = 216.
NMOS 2010 Q5
An(1 h) + Bo(1 h) --> 1/16.8
An (1 h) + Ch(1 h) --> 1/21
Bo(1 h) + Ch(1 h) --> 1/28
Bo(1 h) - Ch(1 h) --> 1/16.8 - 1/21 = 1/84
2 Bo(1 h) --> 1/84 + 1/28 = 1/21
Bo(1 h) --> 1/2 x 1/21 = 1/42
1/42 wall --> 1 h
1 wall --> 42 x 1 = 42 h ---- B
An(1 h) --> 1/16.8 - 1/42 = 1/28
1 wall --> 28 x 1 = 28 h ----- A
Ch(1 h) --> 1/28 - 1/42 = 1/84
1 wall --> 84 x 1 = 84 h ---- C
A + B + C = 28 + 42 + 84
= 154 h
NMOS 2010 Q6
To walk on the same side, Peter would need to walk 150 m more than Queenie first.
50 – 47 = 3 m/min
3 m --> 1 min
150 m --> 150/3 x 1 = 50 min
50 m/min x 50 min = 2500 m
2500 / 300 = 8 r 100 m
To be on the same side, Peter would need to travel another 200 m more. [since he was already 100 m on one side while Queenie was 300 m ahead (i.e. 100 m on another side of the triangle]
200 m / 50 m/min = 4 min
50 + 4 = 54 min
NMOS 2010 Q7
1/2 x 2 x 6 = 6
1/2 x 3 x 1 = 1.5
1/2 x 2 x 4 = 4
1/2 x 7 x 1 = 3.5
6 + 1.5 + 4 + 3.5 = 15
5 x 2 = 10
2 x 1 = 2
4 x 3 = 12
4 x 4 = 16
10 + 2 + 12 + 16 = 40
40 - 15 = 25 cm 2.
NMOS 2010 Q8
Shift triangle ADE to CDF (2 sides same and right angle)
16 = 4 x 4 DEBF is a square
½ x 4 x 2 = 4
16 – 4 = 12 cm²
NMOS 2010 Q9
Q9. a, b, c and d are four distinct positive integers such that the product of any two of them is a multiple of 12. Find the least possible value of a+b+c+d.
2 x 6, 2 x 12, 2 x 18 --> 2 + 6 + 12 + 18 = 38
a + b + c + d = 38
2 x 6, 2 x 12, 2 x 18 --> 2 + 6 + 12 + 18 = 38
a + b + c + d = 38
NMOS 2010 Q10
Q10. A shop owner sold only red dresses and blue dresses. The number of red dresses sold on Tuesday was 10% more than that sold on Monday. The number of blue dresses sold on Tuesday was 10% less than that sold on Monday. The total number of dresses sold on Tuesday was 5% more than that sold on Monday. What percentage of the dresses sold on Monday was red?
M --> 10 R + 10 B = 100%
T --> 11 R + 9 B = 105%
90 R + 90 B --> 900%
110 R + 90 B --> 1050%
20 R --> 1050% - 900% = 150%
10 R --> 10/20 x 150% = 75%
M --> 10 R + 10 B = 100%
T --> 11 R + 9 B = 105%
90 R + 90 B --> 900%
110 R + 90 B --> 1050%
20 R --> 1050% - 900% = 150%
10 R --> 10/20 x 150% = 75%
NMOS 2010 Q11
Q11. When each of the numbers 2010, 2377 and 3111 is divided by a number D, they give the same remainder R. Find the largest possible value of D+R.
2377 – 2010 = 367 -- D
2010 / 367 = 5 R 175
2377 / 367 = 6 R 175
3111 / 367 = 8 R 175
D + R = 367 + 175 = 542
2377 – 2010 = 367 -- D
2010 / 367 = 5 R 175
2377 / 367 = 6 R 175
3111 / 367 = 8 R 175
D + R = 367 + 175 = 542
NMOS 2010 Q12
Q12. We define the ending zeroes of an integer as the number of zeroes at the end of the integer. For example, 2010 has one ending zero whereas 10010000 has four ending zeroes. How many ending zeroes are there when the value of 16x17x18x…..x209x210 is simplified?
25, 75, 175 = ..00 --> 2 x 3 = 6
125 = ..000 --> 3
35, 45, 55, 65, 85, 95, 105, 115, 135, 145, 155, 165, 185, 195, 205 --> 15
50, 150 = ..00 --> 2 x 2 = 4
100, 200 --> 2 x 2 = 4
20, 30, 40, 60, 70, 80, 90, 110, 120, 130, 140, 160, 170, 180, 190, 210 --> 16
6 + 3 + 15 + 4 + 4 + 16 = 48 zeros
25, 75, 175 = ..00 --> 2 x 3 = 6
125 = ..000 --> 3
35, 45, 55, 65, 85, 95, 105, 115, 135, 145, 155, 165, 185, 195, 205 --> 15
50, 150 = ..00 --> 2 x 2 = 4
100, 200 --> 2 x 2 = 4
20, 30, 40, 60, 70, 80, 90, 110, 120, 130, 140, 160, 170, 180, 190, 210 --> 16
6 + 3 + 15 + 4 + 4 + 16 = 48 zeros
NMOS 2010 Q13
a + c = 2 b
b + (b + c) + (a + b) = 180
5 b = 180
b = 180/5 = 36
a + c = 2 x 36 = 72
72 + 36 = 108 degree
NMOS 2010 Q14
Q14. The ratio of male students to female students in a primary school is 5 : 4. The ratio of students who wear spectacles to students who do not is 11:1. Given that 64% of the students who do not wear spectacles are female, find the percentage of female students who do not wear spectacles.
M : F = 5 : 4 = 20 : 16
S : N = 11 : 1 = 33 : 3
64/100 x 3 = 1.92 u
1.92/16 x 100% = 12% -- Female who do not wear spectacles.
M : F = 5 : 4 = 20 : 16
S : N = 11 : 1 = 33 : 3
64/100 x 3 = 1.92 u
1.92/16 x 100% = 12% -- Female who do not wear spectacles.
NMOS 2010 Q15
Q15. a, b, c and d are letters that represent four different positive integers such that axa+bxb=cxc+dxd. Find the least possible value of axa+bxb.
a x a + b x b = c x c + d x d
a x a – c x c = d x d – b x b
(a + c) x (a - c) = (d + b) x (d - b)
(8 + 7) x (8 - 7) = (4 + 1) x (4 - 1)
8 x 8 + 1 x 1 = 7 x 7 + 4 x 4 = 65
a x a + b x b = c x c + d x d
a x a – c x c = d x d – b x b
(a + c) x (a - c) = (d + b) x (d - b)
(8 + 7) x (8 - 7) = (4 + 1) x (4 - 1)
8 x 8 + 1 x 1 = 7 x 7 + 4 x 4 = 65
NMOS 2010 Q16
Q16. There are five members in John’s family: Father, Mother, John, elder sister Helen, and younger sister Lucy. Just before Lucy was born , the average age of the family was 19 years old, and the age of John’s father is the same as the sum of the age of the rest of the family. Two years ago, the average age of the family was 35.2 years old, and the age of John’s mother was eight years more than the sum of the ages of John and Lucy. The current average age of the family, excluding Helen, is 39.5 years old. Find the present age of John.
F, M, H, J, L
L = 0, F + M + J + H = 19 x 4 = 76
F = M + J + H = 76 / 2 = 38
2 years ago, F + M + J + H + L = 35.2 x 5 = 176
M = J + L + 8
Now, F + M + H + J + L = 176 + 5 x 2 = 186
M = J + L + 8 – 2 = J + L + 6
186 – 76 = 110
110 / 5 = 22 years – L
F = 38 + 22 = 60
F + M + J + L = 39.5 x 4 = 158
M + J = 158 – 60 – 22 = 76
M = J + 22 + 6 = J + 28
J = (76 – 28)/2 = 24 years old
F, M, H, J, L
L = 0, F + M + J + H = 19 x 4 = 76
F = M + J + H = 76 / 2 = 38
2 years ago, F + M + J + H + L = 35.2 x 5 = 176
M = J + L + 8
Now, F + M + H + J + L = 176 + 5 x 2 = 186
M = J + L + 8 – 2 = J + L + 6
186 – 76 = 110
110 / 5 = 22 years – L
F = 38 + 22 = 60
F + M + J + L = 39.5 x 4 = 158
M + J = 158 – 60 – 22 = 76
M = J + 22 + 6 = J + 28
J = (76 – 28)/2 = 24 years old
NMOS 2010 Q18
Q18 Albert had a basket of fruits which contained 35 cherries and 50 strawberries, while Calvin had another basket of fruits which contained 45 cherries and 10 strawberries. Albert gave Calvin some cherries and strawberries so that eventually, 40% of Albert’s fruit basket contained cherries and 30% of Calvin’s fruit basket contained strawberries. What was the total number of cherries and strawberries that Albert gave to Calvin?
A --> 35 C + 50 S
C --> 45 C + 10 S
3 x (35 – X) --> 2 x (50 – Y)
105 – 3 X --> 100 – 2 Y
3 X – 2 Y --> 105 – 100 = 5
7 x (10 + Y) --> 3 x (45 + X)
70 + 7 Y --> 135 + 3 X
7 Y – 3 X --> 135 – 70 = 65
5 Y --> 5 + 65 = 70
Y --> 70/5 = 14
3 X --> 5 + 2 x 14 = 33
X --> 33/3 = 11
X + Y --> 11 + 14 = 25 cherries and strawberries.
A --> 35 C + 50 S
C --> 45 C + 10 S
3 x (35 – X) --> 2 x (50 – Y)
105 – 3 X --> 100 – 2 Y
3 X – 2 Y --> 105 – 100 = 5
7 x (10 + Y) --> 3 x (45 + X)
70 + 7 Y --> 135 + 3 X
7 Y – 3 X --> 135 – 70 = 65
5 Y --> 5 + 65 = 70
Y --> 70/5 = 14
3 X --> 5 + 2 x 14 = 33
X --> 33/3 = 11
X + Y --> 11 + 14 = 25 cherries and strawberries.
NMOS 2010 Q19
Q19. A, B and C are letters that represent different odd digits such that ABC is a 3-digit number. If ABC is a multiple of A, B and C , how many different 3-digit numbers ABC are there?
ABC = A x = B x = C x
A, B, C – odd digits
Odd digits – 1, 3, 5, 7, 9
5 x 4 x 3 = 60 different numbers
Numbers that meet the above conditions are
135 = 1 x 135, 3 x 45, 5 x 27
175 = 1 x 175, 7 x 25, 5 x 35
315 = 3 x 105, 1 x 315, 5 x 63
735 = 7 x 105, 3 x 245, 5 x 147
There are 4 different 3-digit numbers.
ABC = A x = B x = C x
A, B, C – odd digits
Odd digits – 1, 3, 5, 7, 9
5 x 4 x 3 = 60 different numbers
Numbers that meet the above conditions are
135 = 1 x 135, 3 x 45, 5 x 27
175 = 1 x 175, 7 x 25, 5 x 35
315 = 3 x 105, 1 x 315, 5 x 63
735 = 7 x 105, 3 x 245, 5 x 147
There are 4 different 3-digit numbers.
NMOS 2010 Q20
Q20 Twenty students took a test worth 100 marks. All their results were positive integers and none of them had the same score. Their teacher ranked them from the highest score (1st) to the lowest score (20th). The top 10 students had an average of 92 marks and the next 10 students have an average of 80 marks. The top 5 students had an average that was 12 marks higher than average of the next 15 students. Find the lowest possible score of the student in the 20th rank.
92 --> 91, 90, 89, 88, 87 (lowest)
93, 94, 95, 96, 97
80 --> 86, 85, 84, 83, 82, 81, 80, 79, 78, ? (lowest)
86 + 85 + 84 + 83 + 82 + 81 + 80 + 79 + 78 = 738
80 x 10 = 800
800 - 738 = 62 (lowest)
93 + 94 + 95 + 96 + 97 = 475
475 / 5 = 95
91 + 90 + 89 + 88 + 87 = 445
800 + 445 = 1245
1245 / 15 = 83
95 – 83 = 12 (as given in question)
So lowest possible score is 62.
92 --> 91, 90, 89, 88, 87 (lowest)
93, 94, 95, 96, 97
80 --> 86, 85, 84, 83, 82, 81, 80, 79, 78, ? (lowest)
86 + 85 + 84 + 83 + 82 + 81 + 80 + 79 + 78 = 738
80 x 10 = 800
800 - 738 = 62 (lowest)
93 + 94 + 95 + 96 + 97 = 475
475 / 5 = 95
91 + 90 + 89 + 88 + 87 = 445
800 + 445 = 1245
1245 / 15 = 83
95 – 83 = 12 (as given in question)
So lowest possible score is 62.
Subscribe to:
Posts (Atom)